Specimen Geometry and Size Effects on the Creep Crack Growth Behaviour of P91 Weldments
S. Maleki
TWI Ltd
A. Mehmanparast, K. M. Nikbin
Imperial College London
M. Yatomi
IHI Corporation
Paper presented at 2013 ASME Pressure Vessels and Piping Conference. Paris, France, 14-18 July 2013.
Abstract
The influence of specimen size and geometry on the creep crack growth (CCG) behaviour of P91 parent and weld materials at 600-625 °C has been examined. CCG tests have been performed on compact tension, C(T), specimens with an initial crack located in the heat affected zone (HAZ). Further tests have also been performed on specimens made of as-received parent material (PM). Higher creep crack growth rates have been found in the HAZ material compared to the PM when the CCG rate is characterized using C* fracture mechanics parameter. The experimental data from these tests are compared to those of available from specimens with different size and geometries. The results are discussed in terms of specimen geometry and constraint effects on the CCG behaviour of P91 weldments at elevated temperatures.
| α, α0 |
Crack length, initial crack length |
| Δa |
Increment of crack growth |
| B |
Specimen thickness |
| Bn |
Net specimen thickness between side-grooves |
| C* |
Steady state creep characterizing parameter |
| D |
Material coefficient in å correlation with C* |
| E |
Elastic (Young’s) modulus |
| E' |
Effective elastic modulus ( = E plane stress or E/(1 v2) for plane strain) |
| H |
Geometry and material function in C* relation |
| K |
Stress intensity factor |
| n |
Power-law creep stress exponent |
| P |
Load |
| t, tf |
Time, test duration |
| tT |
Transition time to widespread creep conditions |
| ν |
Poisson’s ratio |
| W |
Specimen width |
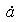 |
Crack growth rate |
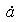 S S |
Crack growth rate under steady state creep conditions |
| η |
Geometry function in C* relation |
 c c |
Component of displacement rate directly associated with the accumulation of creep strains |
 i i |
Component of displacement rate directly associated with instantaneous (elastic and plastic) strains |
 i e i e |
Component of displacement rate directly associated with instantaneous elastic strains |
| σ |
Stress |
| ϕ |
Exponent in correlation of creep crack growth rate with C* |
 T T |
Total load line displacement rate |
Introduction
An important issue to be understood in the life assessment of power plant components is the influence of fabrication processes such as welding on the creep deformation and crack growth behaviour of the material at elevated temperatures. When components start to operate at high temperatures, defects and small cracks are most likely to exist in the heat affected zones (HAZ) subsequent to the welding process [1]. Therefore, it is essential to characterize the creep behaviour of HAZ material in order to estimate the lifetime of components and operate them with suitable safety margins against failure at elevated temperatures.
P91 is widely used in power plant components e.g. thick walled pipes. The creep crack growth (CCG) behaviour of P91 parent material (PM) and weldments has been investigated by a number of researchers e.g. [2-6]. It has been shown in these previous studies that material conditions and specimen size and geometry influence the creep deformation and crack growth behaviour of P91 at the range of temperatures examined. To investigate these effects further, CCG tests have been performed on compact tension, C(T), specimens with an initial crack located in the PM or the boundary between PM and HAZ regions. The results from these tests are compared to the data on specimens with different size and geometries available in the literature [7, 8].
Creep deformation and crack growth relations
Crack Growth
For power-law creeping materials under steady state conditions, the creep strain rate,
, equivalent stress, σ , relation may be written as
where n and A are the power-law creep stress exponent and coefficient, respectively. At long times, where a steady state of creep deformation and damage has developed at a crack tip, the CCG rate,
(or da/dt), may be described by the crack tip parameter C* according to the power law relationship,
where D and ϕ are material constants, which may be temperature and stress state dependent [9], and
vs. C* data appear as a straight line on log-log axes [9]. Prior to steady state conditions being achieved, data points will appear as a ‘tail’ on the
vs. C* plot.
For situations where the crack tip deformation is predominantly elastic (e.g. short times and/or high crack velocity) it is expected that crack growth will be controlled by the linear elastic stress intensity factor, K. Under such conditions, the rate of crack growth can be represented as,
where D' and m are material constants, which may be temperature and stress state dependent.
Evaluation of the Stress Intensity Factor
The stress intensity factor for a sharp crack can be written as
where σ is the applied stress, a is the crack length and Y(a/W) is a dimensionless shape function. The solution of Y(a/W) for conventional fracture geometries can be found in [10]. For a side grooved compact tension specimen the nominal stress can be defined as
where P is the applied load, W is the width of the sample, B is the specimen thickness and Bn is the net thickness between side grooves. The solution of the shape function for a standard C(T) specimen with a/W ≥ 0.45 can be defined as [11]
where f(a/W) is calculated using
Evaluation of the C* Parameter
The C* creep fracture mechanics parameter can be determined experimentally from the load line displacement rate measurements,
, using the relation [12]
where P is the applied load, Bn is the net thickness between side grooves, W is the width, a is the crack length, H and η are geometry dependent constants. For a C(T) specimen H = and η = 2.2 according to ASTM E1457 [12].
Load Line Displacement Rate Measurements
The total load line displacement rate,
in a creep crack growth test can be partitioned into an instantaneous part,
, and a time-dependent part which is directly associated with the accumulation of time dependent creep strains,
The instantaneous displacement rate,
can be further divided into an elastic and a plastic part. The plastic components is generally neglected [12], and the elastic component calculated using [12]
is the crack growth rate, K is the stress intensity factor and E'́ is the effective elastic modulus (E/(1 - v2) for plane strain and E for plane stress conditions).
Validity Criteria
Validity criteria are specified in [12] for the use of the C* parameter to describe creep crack initiation and growth. These are that the transition time, tT, from an elastic crack tip field to a C* controlled creep crack tip field should be exceeded, which may be estimated using [12]
where ‘max’ refers to the maximum value of the term in brackets, which is evaluated for each point in the data set. Also, data points obtained prior to a crack extension Δa of 0.2 mm should be excluded as they are considered to be within the transient crack growth regime where damage is building up to a steady state at the crack tip. In addition, it is necessary to identify the material behaviour as ‘creep-ductile’. Data are considered to be in the creep-ductile regime if the creep load line displacement rate, calculated using Eqns (9) and (10), constitutes at least half of the total load line displacement i.e.
Creep-brittle situations are considered to exist when
Test Details
Specimen Preparation
C(T) specimens were manufactured from an ex-service P91 material provided by IHI Corporation in Japan. The starter crack, introduced into the material using and EDM notch, was located in PM or the boundary region between PM and HAZ. Due to the material availability, the width of W = 32 mm was chosen for the C(T) specimens. All other specimen dimensions are detailed in Table 1. Two PM (denoted PM1-CT32 and PM2-CT32) and three HAZ (denoted HAZ1-CT32, HAZ2-CT32 and HAZ3-CT32) specimens were tested in total. All creep crack growth tests were performed at 600 °C. As seen in Table 1, the normalised initial crack length, a0/W, was 0.45 in all specimens, which is within the valid range specified in ASTM E1457.
Loading Conditions
The loading conditions and a summary of the CCG test results are detailed in Table 2. As seen in this table, lower loads were applied on HAZ specimens compared to the PM because higher creep crack growth rates are generally expected in HAZ material. Also as indicated in Table 2, after a significant amount of time the crack initiation didn’t occur in PM2-CT32 specimen which was initially loaded at a lower level, thus the load was incrementally increased to 8.0 kN in this test. This explains why the test duration, tf, in PM2-CT32 is longer than PM1-CT32, though a higher load was applied on PM2-CT32. Also shown in Table 2 are the values of stress intensity factor at initial crack length, K(a0), crack extension, Δa, normalised transition time, tT/tf , and normalised initiation time, t0.2/tf, for all the CCG tests examined.
CREEP CRACK GROWTH CHARACTERIZATION IN PARENT AND HAZ MATERIALS
Crack Growth Behaviour
The variation of the load line displacement, Δ, and the crack length, a, normalised by the specimen width, W, are plotted against time normalised by the test duration, tf, in Figure 1 and Figure 2, respectively. As seen in these figures, rapid increase in the load line displacement rate and crack growth rate is observed towards the end of the tests in all the specimens examined. Note that the rate of change in the load line displacement and creep crack length at the end of the test depends on the point at which the test is interrupted. As seen in Figure 2, at a given normalised time the normalised crack length measured on HAZ specimens is generally larger than those of obtained from PM specimens. Also seen in Figure 2 and Table 2 is that the total creep crack extension in HAZ specimens is greater than the ones measured on PM samples.
Creep Crack Growth Rate Variation against Stress Intensity Factor
The crack growth rates have been correlated with the stress intensity factor, K, in Figure 3. As seen in this figure, no particular trend can be deduced when the creep crack growth rate, da/dt, is correlated with K. This may indicate that the elastic displacement rate is insignificant in these tests and thus the material cannot be considered as creep-brittle and characterised using the stress intensity factor. Therefore, the creep crack data are correlated with the C* steady state creep fracture mechanics parameter next and the trends are examined.
C* Validity Criteria
The C* validity criteria specified in ASTM E1457 have been examined and the results are shown in Figure 4 where the variation of the creep to total load line displacement rate is plotted against the normalised time. The data points corresponding to Δa < 0.2, t < tT and
are considered invalid and shown in grey symbols in Figure 4. As seen in this figure, the creep to total load line displacement rate is in the range of 0.85
for the PM specimens examined. The
values are generally high in the HAZ specimens, however a significant drop can be observed in the creep to total load line displacement rate data for HAZ2-CT32 and HAZ3-CT32 specimens after approximately 80% of their test duration, though the values are still above 0.5. It can be observed in Figure 4 that although all the data points from the CCG tests on the P91 PM and HAZ materials can be considered as creep-ductile i.e.
trends in PM samples are generally greater than the HAZ which indicates that the contribution of elastic term (i.e. see Eqns (9) and (10)) in the load line displacement rate is less significant in parent material compared to HAZ.
Creep Crack Growth Data Analysis
The creep crack growth rate data from all the tests examined on PM and HAZ materials are correlated with the C* fracture mechanics parameter in Figure 5. It has been noted that although only the valid data points from Figure 4 are included in this figure, some tails are still apparent particularly in the CCG data from HAZ specimens. This implies that applying the ASTM C* validity criteria don’t necessarily remove the entire tail region in da/dt vs. C* trends.
It can be observed in Figure 5 that the CCG trend obtained from PM1-CT32 is consistent with that of obtained from PM2-CT32 sample. Also seen in Figure 5 is that for a given value of C*, a higher creep crack growth rate is observed in the HAZ specimens compared to the PM. In order to quantify the amount of increase in the CCG rate of the HAZ material compared to the PM, the empirical creep crack growth power law constants (i.e. see Eqn (2)) have been obtained from the line of best fits to the valid data points and are summarised in Table 3. As seen in Table 3, similar slopes of close to unity have been found in the CCG behaviour of the HAZ and PM specimens. Also seen in Table 3 and Figure 5 is that for a given value of C*, the creep crack growth rate in the HAZ data is around 8 times higher than the PM data.
Specimen geometry and size effects
Comparison to the Existing Parent Material Data
The CCG data on PM1-CT32 and PM2-CT32 parent material specimens tested at 600 °C are compared to those of available at 600─625 °C on C(T) specimens of width W = 25 and 50 mm (with B/W = 0.5) taken from [7, 8] and also to the existing test data on single edge notch tension , SENT, specimens in Figure 6. As seen in this figure, the CCG test data on C(T) specimens with W = 32 mm generally fall close to or below the lower bound of the experimental data scatter available for the parent material at 600─625 °C. It can be observed in Figure 6 that for the range of temperatures examined, the CCG trend from C(T) specimens with W = 50 mm, is higher than those of obtained from SENT samples and also C(T) specimens with W = 25 and 32 mm. Also seen in Figure 6 is that the data scatters are similar for C(T) specimens with W = 25 mm and SENT samples. The available CCG data scatter bands from these tests, which are relatively wider than those of obtained from other specimens, generally fall on top of or in between the CCG trends for C(T) specimens with W = 50 mm (which have the highest CCG rates) and W = 32 mm (which show the lowest CCG rates).
Comparison to the Existing HAZ Material Data
The valid CCG data points from the tests on HAZ1-CT32, HAZ2-CT32 and HAZ3-CT32 at 600 °C are compared to the available experimental data on HAZ material C(T) specimens with W = 25 mm tested at 600─625 °C taken from [7, 8], in Figure 7. As seen in this figure, the CCG trends observed from C(T) HAZ specimens with the width of 25 and 32 mm are similar within the scatter, if the tails in the HAZ-CT32 tests are not considered in comparisons.
Discussions and conclusions
A higher creep crack growth rate is observed in HAZ specimens compared to the parent material when the data are correlated with the C* fracture mechanics parameter. This is thought to be due to the lower creep ductility and also higher yield stress in HAZ material which limits the plasticity effects in the CCG behaviour of the material and thus leads to higher creep crack growth trends.
Some discrepancy can be observed in da/dt vs. C* trends from the tests on PM specimens with different sizes and geometries at 600─625 °C. As shown in Figure 6, a higher CCG trend in parent material was observed in C(T) specimens of width W = 50 mm (and B = 25 mm) compared to those of W = 25 and 32 mm (and B = 12.5 mm). This can be due to the higher specimen constraint level in larger C(T) specimens which leads to dominant plane strain conditions in the samples and thus higher creep crack growth rates. The lowest CCG trend was seen in C(T) PM specimens of width W = 32 mm. Note that although PM1-CT32 and PM2-CT32 C(T) specimens of W = 32 mm had a different width compared to the C(T) specimens with W = 25 mm, but the total thickness, B, was the same in these samples (i.e. B = 12.5 mm). It may explain why the CCG data for CT32 specimens fall close to the scatter band of CT25 specimens. Note that all the CCG data on parent material C(T) specimens of width 25 mm are for 625 °C, thus a slight drop in the CCG rate of CT32 specimens performed at 600 °C may be related to the temperature effects. Therefore, repeating the tests on C(T) specimens with W = 25 mm at 600 °C may result in CCG trends closer to that of obtained from C(T) specimens with W = 32 mm tested at the same temperature.
Figure 6 o shows that the specimen geometry can influence the CCG behaviour of P91 parent material. This is because of the difference between specimen constraint levels in various geometries. For the given material and testing temperature, similar CCG trends in SENT and CT25 samples may be due to similar levels of specimen constraint in the examined test pieces.
The comparison of the available creep crack growth data on HAZ material C(T) specimens with W = 25 at 600─625 °C to those of width W = 32 mm at 600 °C shows that similar trends can be observed in all HAZ samples, if the tails remained in the data subsequent to C* validity criteria being applied are excluded. This can be due to the same specimen thickness (i.e. B = 12.5 mm) and thus similar constraint levels in C(T) HAZ specimens with W = 25 and 32 mm. Further tests on C(T) specimens of width W = 32 made of parent material and HAZ are needed to confirm the observed trends.
Acknowledgments
Discussions with Dr. Catrin M. Davies of Imperial College London are greatly acknowledged. The authors also would like to thank Mr Oliver Choon and Hyung Roh formerly of Imperial College London, towards the preparation of experimental data.
References
- Toft, L. and Yeldham, D., Weld performance in high pressure steam generating plant in the Midlands Region CEGB., in Proceedings of the Conference on Welding Research Related to Power Plant. 1972: Southampton, UK.
- Dogan, B. and Petrovski, B., Creep crack growth of high temperature weldments, International Journal of Pressure Vessels and Piping, 2001, 78(11-12), pp. 795-805.
- Tan, M., Célard, N. J. C., Nikbin, K. M and Webster, G. A., Comparison of Creep Crack Initiation and Growth in Four Steels Tested in HIDA, International Journal of Pressure Vessels and Piping, 2001, 78(12), pp. 737-747.
- Le Mat Hamata, N. a. S., I. A., Creep Crack Growth of Seam-Welded P22 and P91 Pipes with Artificial Defects. Part I: Experimental Study and Post-Test Metallography, International Journal of Pressure Vessels and Piping, 2001a, 78(11-12), pp. 819-826.
- Le Mat Hamata, N. a. S., I. A., Creep Crack Growth of Seam-Welded P22 and P91 Pipes with Artificial Defects. Part II: Data Analysis, International Journal of Pressure Vessels and Piping, 2001b, 78(11-12), pp. 827-835.
- Hyde, T. H., Saber, M. and Sun., W., Testing and modelling of creep crack growth in compact tension specimens from a P91 weld at 650° C, Engineering fracture mechanics, 77(15), pp. 2946.
- European Commission, Validation Expansion and Standidarzation of Procedures for High Temperature Defect Assessment (HIDA), 1995.
- CRETE, Development and Harmonisation of Creep Crack Growth Testing for Industrial Specimens - A Route to a European Code of Practice, EU Framework 5 Project, GRD2-2000-30021, 2001-2004.
- Webster, G. A. and Ainsworth, R. A., High Temperature Component Life Assessment, 1st ed, 1994, London: Chapman and Hall.
- Anderson, T. L., Fracture Mechanics: Fundamentals and Application, 1991, Boston: CRC Press.
- Tada, H., Paris, P. C. and Irwin, G. R., The Stress Analysis of Cracks Handbook, 1985, Saint Louis: Paris Productions & (Del Research Corp.).
- ASTM, E1457-07: Measurement of Creep Crack Growth Rates in Metals, in Annual Book of ASTM Standards, Vol. 03.01, 2007, ASTM International, pp. 1012-1035.
Tables
Table 1: C(T) specimen geometries
| Specimen ID |
Material Condition |
W(mm) |
B(mm) |
Bn (mm) |
a0/W(mm) |
| PM1-CT32 |
PM |
32.0 |
12.5 |
10.3 |
0.45 |
| PM2-CT32 |
PM |
32.0 |
12.5 |
10.3 |
0.45 |
| HAZ1-CT32 |
HAZ |
32.0 |
12.5 |
10.3 |
0.45 |
| HAZ2-CT32 |
HAZ |
32.0 |
12.5 |
10.3 |
0.45 |
| HAZ3-CT32 |
HAZ |
32.0 |
12.5 |
10.3 |
0.45 |
Table 2: Loading conditions and a summary of CCG test results
| Specimen ID |
P (kN) |
K(a0 ) (MPa√m) |
tf (h) |
Δa (mm) |
|
|
| PM1-CT32 |
6.2 |
25.4 |
620 |
1.0 |
2.3 |
12.9 |
| PM2-CT32 |
8.0* |
32.7 |
1138 |
1.7 |
26.5 |
66.0 |
| HAZ1-CT32 |
4.0 |
16.4 |
1434 |
2.6 |
0.8 |
29.1 |
| HAZ2-CT32 |
5.7 |
23.3 |
797 |
3.1 |
2.6 |
1.8 |
| HAZ3-CT32 |
5.7 |
23.3 |
483 |
4.0 |
1.5 |
1.4 |
Table 3: Empirical creep crack growth power law constants
| Material |
D |
ϕ |
| PM |
10.0 |
0.99 |
| HAZ |
83.5 |
0.98 |
Figures
Figure 1: Variation of load line displacement against time
Figure 2: Variation of creep crack extension against time
Figure 3: Correlation of the creep crack growth rates with the stress intensity factor
Figure 4: Analysis of the validity criteria for correlating the CCG data with the C* parameter
Figure 5: Correlation of the creep crack growth rates with the C* fracture mechanics parameter
Figure 6: Comparison of the parent material CCG data for a range of specimen size and geometries
Figure 7: Comparison of the HAZ material CCG data for C(T) specimens with different dimensions